Hyperbolic space (H3) is 3D analogue of well-known hyperbolic (or Lobachevskian) plane. It can be divided to regular polyhedra (cells) - finite or infinite - in many ways. Some of tilings may be colored by finite number of colors in periodic patterns.

Once you have periodic pattern in H3, you may use it to make twisty puzzle: cut each cell with surfaces, "parallel" to its faces, and glue parts ("stickers") of adjacent cells together. Twist of such puzzle is the rigid motion of the cell and all its adjacent stickers.

In the program one particular space division is used. It has Schlafli symbol {6,3,3} that means that boundary of cell consists of hexagons, three hexagons meet in the vertex of boundary and three cells meet in every edge of the cell. So boundary of the cell is infinite itself - it looks like regular hexagonal tiling of Cartesian plane. Periodic pattern in H3 derives some pattern on the boundary, and it every sticker seems to be repeated infinite number of times.

In the solved position every cell has stickers of the same color:

This picture shows view from the wide gap between the central sticker of some cell (it's invisible from this position) and its boundary stickers. You can see periodic pattern of central sticker of other cells. Small particles in the yellow cell are its own boundary stickers, and so on - the space has infinite depth.

There is an alternative view of the same space:

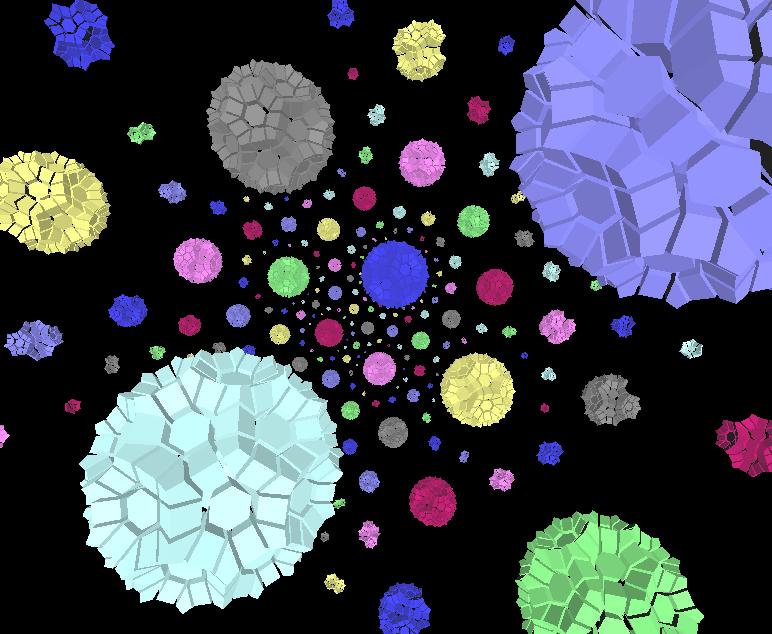
Here space between stickers is small, but cells are shrinked so you can see many of them. Cells look like balls (made of hexagons!), but in reality they are extending to the infinity behind their visible part.

There are two views of the scrambled puzzle:

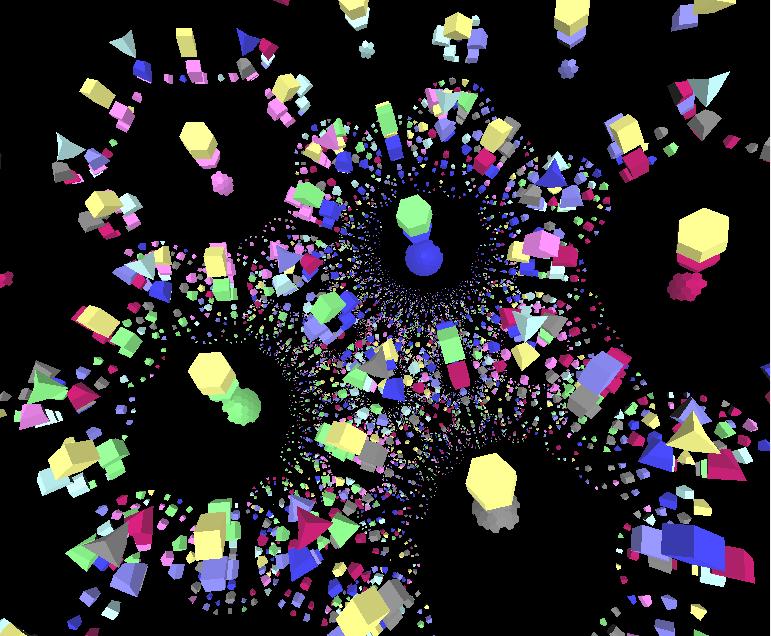
Actually, puzzle in the second picture is partially solved - all 2-Color pieces are in place. You can select size of cells and stickers as you wish using the control panel. For the twist just click (or right-click) some sticker. Also you can change colors, hide some stickers or pieces, create and use macros, and so on.

The program (zip with executable file) is here. Unpack it in one folder and double-click the .exe file to run program. It requires Microsoft .NET 2.0. Now program works in Windows only (and there are no plans to convert it for Linux). Instruction for the puzzle (commands description) is here

There are 7 different puzzles implemented - from 8 to 52 colors. Only one of them was solved (12 colors) in the beta version of program, and no solves in the release version were made yet. So solver lists are empty and awaiting for you.
UPD: 8 Colors is solved...

Good luck!

Andrey

Magic 3D Hyperbolic Tile puzzle took many ideas from MagicCube4D project. I would like to thank its developers Don Hatch, Melinda Green, Jay Berkenbilt and Roice Nelson for this wonderfull puzzle and for the very interesting 4D Cubing Group! Special thanks to Roice Nelson for his Magic Tile program that is the first puzzle in the Hyperbolic Plane!

Some links:

• Magic Tile - equivalent to Rubik's Cube in Hyperbolic plane
• MagicCube4D - Not only cubes. There are many other 4D puzzles inside one program, most of them are still unsolved
• MagicCube5D - 5-dimensional magic cubes from 2⁵ to 7⁵.
• MagicCube7D - seven-dimensional cube! Cubes from 3⁴ to 5⁷ are implemented
• Magic120Cell - 120-Cell is one of two largest regular polytopes in 4D. This puzzle is a 4D analogue of the 3D Megaminx. It has currently been solved exactly once.
• 4D Cubing Group - Mailing list for hypercubists.
• Download - Alternative link to M3dHT633 program

If you have comments, suggestions or log files with solved full scramled puzzles, mail me.